What is Option Premium & How it is Calculated?

Options are flexible financial contracts in the derivatives market. They allow holders to buy/sell underlying assets minus any obligations. Additionally, the option premium is the price at which any option is purchased/sold and is paid by option holders. Yet, there is no obligation on the buyer to exercise the right, while the seller receives the premium upon selling the underlying asset (in case the buyer exercises the right). The option premium determines the profits of both buyers and sellers. Here is a closer look at the concept and its calculation process.
What is Option Premium?
The option premium is the price of the financial contract of the underlying asset for the strike price. The buyer pays the premium to purchase the options contract. The seller’s profit is determined by the premium at which the contract is sold. Due to the dynamic pricing in the derivatives market, the option premium changes with every transaction. Determining the right option premium for the call and put order is crucial to generating profit with an options contract. The generic option premium formula is,
Option Premium = Intrinsic value + Time value + Volatility value
Factors Impacting Premium in Option Trading
Apart from the supply and demand of the options contract, numerous factors affect the price of an option. Crucial factors that influence option premium calculation are:
#1 Intrinsic Value
The intrinsic value is the difference between the option's strike price and the underlying asset's current price. For calculations, the difference between the spot price and the strike price is the intrinsic value. The intrinsic value can be positive or zero. Generally, all in-the-money call and put options have positive intrinsic value.
# 2 Time Value
The excess of the option's price over its intrinsic value is the time value. It is also called extrinsic value. It is the intangible portion of the option value. Depending on the expiration date of the options contract, time is available for the option's price to move in favour of the buyer. Option holders have more chances of making a profit with extended time till expiry as the price can align favourably.
#3 Implied Volatility
Depending on the market conditions, there may be significant price movements. This volatility of the underlying assets also influences the option premium. The higher volatility results in a premium increase, thus making the option more valuable.
# 4 In-the-Money Status
The relationship of the option to the underlying asset also influences the premium. In-the-money options generally have higher premiums due to the higher demand in the market. Out-of-the-money options have lower premiums.
#5 Time Until Expiration
The duration until the expiration date of the option also affects the premium. Options with more extended expiration periods carry higher premiums. This is because of the availability of time for the options to move favourably.
#6 Interest
The prevailing interest rates also impact the option premium due to the opportunity cost of the tying up of capital. Higher rates result in an increase in the put option premium and a decrease in the call option premium. Similarly, lower interest rates have the opposite effect.
Special Considerations for Options Pricing
Here are a few considerations for options pricing, i.e. the factors that determine it.
Underlying Asset Price
The worth of any asset under the options contract will estimate the worth of the option. It results from the intrinsic value of the option or the disparity between the asset’s strike price and market price.
Strike Price
Call options are more valuable when their strike prices are lower than the present stock prices. This allows you to purchase the stock at a better rate. For put options, a higher strike increases the value of the option. It enables you to sell the stock at a higher price.
Time to Expiration
Time to expiration impacts option pricing owing to the time value concept. The closer an option is to the expiration date, the higher the erosion in time value or time decay. Hence, options with longer expiration dates often have higher premiums, enabling more time for favorable movements in prices for the underlying asset.
Volatility
Higher volatility may indicate increased probability of major price swings in the underlying asset. This enhances the chances of options closing in-the-money by expiration. Such unpredictability leads to higher option premiums, with buyers inclined to pay more for unlocking considerable potential gains.
Interest rates
Interest rates directly impact option pricing through the cost of carry. It indicates the opportunity cost involved in tying up capital. In case of call options, higher rates usually increase the price (buying the stock later through exercising the option becomes more tempting owing to possible gains from investing the fund elsewhere). Put options also become less costly, since holding cash becomes more favorable.
Dividends
These impact the price movements of the underlying stock considerably. Whenever a company declares a dividend, its stock price falls by the approximate dividend amount on the ex-dividend date. In the case of call options, this fall makes the option less appealing to lower the value. However, for put options, the fall increases the value of the contract. The lower stock price enhances the overall appeal of selling at the higher strike price.
Black Scholes Method for Calculating Options Pricing
The Black Scholes options pricing model is a specific mathematical system used for computing the prices of European call options. It takes factors like the current price of the underlying security, volatility, time to expiry, and the risk-free interest rate into account. This formula was created in 1973 by Fischer Black and Myron Scholes.
The Black Scholes formula is,
C = S0N (d1) — Ke—rTN (d2)
In this case, the key variables are the following:
- (C) is the call option price
- (S_0) is the present stock price
- (K) is the strike price
- (r) is the risk-free interest rate
- (T) is the time to expiration
- N(d) shows the cumulative distribution function of the standard normal distribution.
To compute (d1) and (d2)
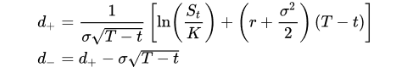
However, note that there are some assumptions upon which the Black-Scholes model operates. These include the following:
- A lognormal distribution is followed by the price of the underlying asset, meaning that it may increase exponentially without falling below zero.
- The volatility of the underlying asset will remain unmoved throughout the life of the option. Yet, the assumption holds no practical relevance in actual market conditions.
- An initial assumption of the model is that the underlying asset does not distribute any dividends throughout the duration of the option. However, some variations like the Black Scholes Merton model account for dividends.
- Markets are efficient, with all known data already accounted for in terms of asset prices.
- There are no chances of arbitrage, thereby guaranteeing the alignment of the option pricing with the value of the underlying asset.
The risk-free interest rate stays fixed and known throughout the lifespan of the option. The Greek letters used are:
- Delta
- Gamma
- Vega
- Theta
- Rho
The following table shows what these Greek letters represent:
|
Greek |
Meaning |
|
Delta |
Measures rate of change of option premium for a 1-point change in the price of the underlying security |
|
Gamma |
Measures the rate of change of Delta for a 1-point change in the price of the underlying security |
|
Vega |
Measures the change in option premium for a 1% change in implied volatility |
|
Theta |
Measures decay in time value for a 1-day change in time till expiry |
|
Rho |
Measures change in option premium for a 1% change in interest rate |
The Black Scholes model enables investors to calculate the fair market value of options considering multiple volatile factors. The systematic approach revolutionised option pricing to estimate the value of financial derivatives. It is an important tool in financial markets.
Conclusion
The option premium is a dynamic pricing influenced by multiple volatile factors. It is a representation of the cost of entering an option contract. Knowing this, investors can understand the potential profit or loss in the derivatives market. You can compare the premiums for different options to make informed decisions.